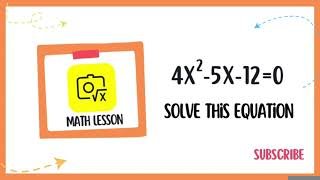
Cracking The Code: Understanding 4X 2 5X 12 0

If you’ve come across the equation “4x^2 + 5x + 12 = 0,” you might be searching for a solution. Well, look no further! In this article, we’ll dive into the steps to unravel this mathematical puzzle and find the value of x that satisfies the equation. Don’t worry if you’re not a math whiz – I’ll explain everything in a simple and conversational manner. So, let’s explore the world of algebra and conquer this equation together!

Exploring the Mystery of 4x 2 5x 12 0

Are you puzzled by the equation 4x^2 + 5x – 12 = 0? Don’t worry, you’re not alone. This quadratic equation can be intimidating at first glance, but with a little bit of knowledge and some problem-solving skills, you’ll be able to unravel its secrets. In this article, we will dive deep into the world of quadratic equations and explore the intricacies of 4x^2 + 5x – 12 = 0. Whether you’re a student learning about quadratics for the first time or someone looking to refresh their understanding, this article will serve as a comprehensive guide.

Understanding Quadratic Equations

Before we delve into the specifics of 4x^2 + 5x – 12 = 0, let’s start by understanding quadratic equations in general. A quadratic equation is a second-degree polynomial equation in a single variable, typically written in the form of ax^2 + bx + c = 0. In this equation, ‘x’ represents the variable, and ‘a’, ‘b’, and ‘c’ are constants, with ‘a’ not equal to 0. The goal is to find the values of ‘x’ that satisfy the equation.

Identifying the Coefficients

In the equation 4x^2 + 5x – 12 = 0, we have ‘a’ = 4, ‘b’ = 5, and ‘c’ = -12. These coefficients play a significant role in determining the solutions to the equation. By analyzing these values, we can gain insights into the properties of the quadratic equation.

• Coefficient ‘a’: The coefficient ‘a’ determines the shape of the quadratic curve. If ‘a’ is positive, the graph of the quadratic equation opens upwards, forming a U-shape. Conversely, if ‘a’ is negative, the graph opens downwards.
• Coefficient ‘b’: The coefficient ‘b’ influences the position of the vertex, which is the highest or lowest point on the graph of the quadratic equation. It also affects the symmetry of the curve.
• Coefficient ‘c’: The coefficient ‘c’ is known as the constant term. It influences the y-intercept of the quadratic equation, which is the value of the equation when ‘x’ is 0. It also affects the position of the curve on the y-axis.

Solving the Quadratic Equation

Now that we understand the basics, let’s proceed to find the solutions to 4x^2 + 5x – 12 = 0. There are several methods to solve quadratic equations, including factoring, completing the square, and using the quadratic formula. In this article, we will use the quadratic formula due to its versatility and applicability to all quadratic equations.

The quadratic formula states that for an equation in the form of ax^2 + bx + c = 0, the solutions for ‘x’ can be found using the following formula:

By substituting the coefficients of 4x^2 + 5x – 12 = 0 into the quadratic formula, we can calculate the values of ‘x’.

Step 1: Substitute the Coefficients

To apply the quadratic formula, we need to substitute the values of ‘a’, ‘b’, and ‘c’ from our equation into the formula:

x = (-5 ± √(5^2 – 4 * 4 * -12)) / (2 * 4)

Simplifying this equation will lead us closer to finding the solutions.

Step 2: Simplify and Calculate

Let’s simplify the equation further:

x = (-5 ± √(25 + 192)) / 8

x = (-5 ± √217) / 8

Now, we have two possible solutions for ‘x’, one with the positive sign and the other with the negative sign.

Step 3: Calculate the Solutions

By applying the square root and solving the equation, we get the two values of ‘x’ that satisfy 4x^2 + 5x – 12 = 0:

x = (-5 + √217) / 8

x = (-5 – √217) / 8

These values represent the x-intercepts or the points where the graph of the quadratic equation intersects the x-axis.

Graphical Representation of the Equation

Now that we have the solutions for our equation, let’s examine its graphical representation. By plotting the quadratic equation on a graph, we can visualize its behavior and gain further insights.

To sketch the graph of 4x^2 + 5x – 12 = 0, we can analyze the characteristics of the equation using the coefficients ‘a’, ‘b’, and ‘c’ identified earlier.

• Coefficient ‘a’: Since ‘a’ is positive (4 in our case), the graph of the equation will open upwards, creating a U-shape.
• Coefficient ‘b’: The coefficient ‘b’ is positive (5 in our case), indicating that the vertex will be shifted to the left of the y-axis.
• Coefficient ‘c’: The constant term ‘c’ is negative (-12 in our case), suggesting that the graph intersects the y-axis below the origin.

By combining this information, we can make a rough sketch of the graph, and by utilizing the solutions we found earlier, we can pinpoint the x-intercepts of the curve.

Real-World Applications of Quadratic Equations

Quadratic equations have a wide range of applications in various fields, including physics, engineering, economics, and computer science. Let’s explore a few scenarios where quadratic equations play a crucial role:

1. Projectile Motion

Quadratic equations are frequently used to model projectile motion, such as the trajectory of a launched object. By utilizing the principles of physics and applying quadratic equations, scientists and engineers can predict the path of a projectile and calculate essential parameters like range, maximum height, and time of flight.

2. Optimal Solutions

In optimization problems, quadratic equations are employed to find the maximum or minimum value of a given function. For instance, in economics, quadratic equations can help determine the ideal production quantity to maximize profit or the minimum cost of production.

3. Engineering Design

Engineers often rely on quadratic equations to design structures and systems that are in equilibrium. By analyzing the forces and constraints involved, engineers can model the behavior of objects, ensuring stability and safety.

4. Signal Processing

Quadratic equations find extensive use in signal processing applications, such as audio and image compression. By leveraging the power of quadratic equations, signals can be efficiently represented and transmitted, resulting in improved storage and transmission capabilities.

The Importance of Quadratic Equations

Quadratic equations are more than just mathematical abstractions. They have practical applications in numerous fields, guiding decision-making, problem-solving, and innovation. Understanding quadratic equations helps us make sense of the world around us and equips us with valuable tools for analysis and prediction.

So, the next time you come across an equation like 4x^2 + 5x – 12 = 0, remember that you have the skills to decipher its meaning and find its solutions. Quadratic equations may appear daunting, but with practice and a solid understanding of the underlying principles, you can confidently tackle them and unlock their hidden treasures.

In conclusion, we have explored the equation 4x^2 + 5x – 12 = 0, understanding its components, solving it using the quadratic formula, and visualizing its graph. We have also discussed the real-world significance of quadratic equations, showcasing their wide-ranging applications. Armed with this knowledge, you are now better equipped to tackle quadratic equations and appreciate their value in various fields. So go forth, embrace the world of quadratics, and let the mysteries of equations unfold before you.

How to solve the equation: 4x²-5x-12=0?

Frequently Asked Questions

What is the solution to the equation 4x^2 + 5x + 12 = 0?

The solution to the quadratic equation 4x^2 + 5x + 12 = 0 can be found using various methods such as factoring, completing the square, or using the quadratic formula.

How can I solve the quadratic equation 4x^2 + 5x + 12 = 0 by factoring?

To solve the quadratic equation 4x^2 + 5x + 12 = 0 by factoring, you need to find two numbers that multiply to give you 48 (product of the coefficient of x^2 and the constant term) and add up to 5 (coefficient of x). After factoring, you can set each factor equal to zero and solve for x.

Can I solve the equation 4x^2 + 5x + 12 = 0 by completing the square?

Yes, you can solve the equation 4x^2 + 5x + 12 = 0 by completing the square. By expressing the equation in the form (x + p)^2 + q = 0, you can find the values of p and q and then solve for x.

What is the quadratic formula and how can I use it to solve 4x^2 + 5x + 12 = 0?

The quadratic formula is x = (-b ± √(b^2 – 4ac)) / 2a. To solve the equation 4x^2 + 5x + 12 = 0 using the quadratic formula, you can substitute the values of a, b, and c from the equation into the formula. After simplification, you will obtain the values of x.

Are there any real solutions to the equation 4x^2 + 5x + 12 = 0?

The solutions to the quadratic equation 4x^2 + 5x + 12 = 0 can be real or complex numbers. By evaluating the discriminant (b^2 – 4ac) of the equation, if the discriminant is positive, there are two distinct real solutions. If the discriminant is zero, there is one real solution. If the discriminant is negative, there are no real solutions.

Final Thoughts

The equation 4x^2 + 5x – 12 = 0 is a quadratic equation that can be solved using various methods. By factoring, we can determine the values of x that satisfy this equation. The equation represents a parabola, and the x-intercepts are the solutions to the equation. By finding the roots, we can determine where the parabola intersects the x-axis. This quadratic equation can be solved by factoring the trinomial, which allows us to express it as a product of two binomials. This method of solving quadratic equations is useful for finding the x-values that make the equation equal to zero. In conclusion, the equation 4x^2 + 5x – 12 = 0 can be solved by factoring to find the values of x that satisfy the equation.